ZBORNÍK SYMPÓZIA
O POČÍTAČOVEJ GEOMETRII
SCG´2010
PROCEEDINGS OF SYMPOSIUM
ON COMPUTER GEOMETRY
SCG´2010
Volume 19
Slovak Society for Geometry and Graphics
Mechanical Engineering Faculty
Civil Engineering Faculty
Slovak University of Technology in Bratislava
October 2010, Kočovce, SR
ISBN 978-80-227-3364-9
Contents
Abstracts
Blowing up a Singular Point of Algebraic Curve on
Algebraic Surface
Martina Bátorová1, Pavel Chalmoviansky2
KAGDM FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
1e-mail: martina.batorova
fmph.uniba.sk
2e-mail: pavel.chalmovianskyfmph.uniba.sk
Abstract. The presence of singular points on an algebraic curve implies a different local behaviour.
The structure of a singular point of an algebraic curve is well understood via normalization
of the curve. The standard tool in such a process is the blowup enabling us to find a birationally
equivalent curve, which is either regular, or is less singular in certain way. In the latter
case, we can repeat the process and obtain a regular curve after a finite number of steps. We
present a detailed description of blowup with examples and figures.
Keywords: singular point, algebraic curve, normalization, blowup, -process.
Back
Úlohy o dotykoch v programe GeoGebra
Martin Billich
Pedagogická fakulta KU v Ružomberku, Hrabovská cesta 1, 034 01 Ružomberok,
SR
e-mail: billichku.sk
Abstrakt: V súčasnosti existuje množstvo dynamických geometrických softvérov, ktoré slúžia nielen na rysovanie rôznych geometrických situácií v riešení klasických planimetrických úloh, ale poskytujú študentom ideálne prostredie na vizualizáciu geometrických vzťahov, tvorbu hypotéz a ich overovanie. Tento článok sa zaoberá možnosťou využitia kužeľosečiek ako množín bodov daných vlastností v riešení konštrukčných úloh o dotýkajúcich sa kružniciach v prostredí programu GeoGebra.
Kľúčové slová: GeoGebra, kužeľosečky, množiny bodov daných vlastností
Summary. GeoGebra is one of the most original mathematical tools that joins geometry, algebra and calculus. This open source tool helps students to acquire knowledge about geometric objects. We demonstrate a method in which conics are used as tools in solving tangency problems related to circles. Although the examples given in this paper are related to the parabola, the strategies and methods are also usable to central conics.
Back
Testing Vertical Monotony of Polygons
Miloš Božek
Comenius University, Faculty of Mathematics, Physics and Informatics
Mlynská dolina, 842 48 Bratislava, SR
e-mail: bozekfmph.uniba.sk
Abstract: The aim of the presented paper is to prove some necessary and sufficient condition for an art of monotony of polygons. Consequently, we get an algorithm with linear time
complexity for determining all vertical monotony directions of a polygon. It enables us to
decide whether two given polygons are vertically monotone with respect to a common
direction.
Keywords: Monotone polygon, external angle of a polygon, convex and non-convex vertex of a polygon.
Back
Geometria informačného prieskumu
Eva Capková
KMKD, FPV, ŽU v Žiline, Univerzitná 8215/1, 010 26 Žilina, SR
e-mail: eva.capkovafpv.uniza.sk
Abstrakt: Príspevok prináša pohľad na jeden zo spôsobov využitia geometrie v informačnej vede, konkrétne v informačnom prieskume. Predstavuje zaujímavú publikáciu popredného odborníka Keitha van Rijsbergena:„Geometria informačného prieskumu“, v ktorej spája tri modely informačného prieskumu: logický, vektorový a pravdepodobnostný, a ukazuje, ako sa dajú opísať a reprezentovať v Hilbertovom priestore, pričom geometriu používa ako „jazyk“ vhodný na vyjadrenie týchto modelov informačného prieskumu.
Kľúčové slová: informačný prieskum, modely informačného prieskumu, relevancia, kvantová mechanika, Hilbertov priestor
Summary. This contribution deals with one way of using geometry in information science, particularly in information retrieval. The interesting publication of the leading specialist Keith van Rijsbergen: “The Geometry of Information Retrieval” is introduced, in which the author joins three models of information retrieval: a logical, a probabilistic and a vector space model and shows how they can be described and represented in the Hilbert space. The geometry can be seen as a „language“ for expressing the different models of information retrieval.
Back
Aplikácie matematiky a insitná tvorba
v matematike
Ján Čižmár
Fakulta matematiky, fyziky a informatiky Univerzity Komenského, Mlynská dolina, 842 48 Bratislava, SR
e-mail: jan.cizmarfmph.uniba.sk
Abstrakt: Článok sa zaoberá problémami aplikácií matematiky v matematických aj nematematických disciplínach. Sleduje vzťah medzi bázovou matematickou teóriou a jej modifikáciou v oblasti aplikácie. Zdôrazňuje nevyhnutnosť dôkladnej znalosti aplikovanej teórie. Analyzuje prípad narušenia tejto zásady vedúci k insitnej tvorbe pochybných pojmov.
Kľúčové slová: aplikácia matematiky, bázová teória, oblasť aplikácie, insitná tvorba
Summary. This paper deals with problems of application of mathematics in both mathematical and non-mathematical branches. It investigates a relation between a mathematical theory and its modification in the field of application. It emphasizes a necessity of a profound knowledge of the applied theory. It analyzes a case of violating this principle, that is followed by a dilettantish creation of dubious concepts.
Back
GeoGebra a dynamické pracovné listy
z matematickej analýzy
Janka Drábeková
FEM SPU, Trieda Andreja Hlinku 2, 949 76 Nitra, SR
e-mail: Janka.Drabekovafem.uniag.sk
Abstrakt: Vizualizácia učiva má v matematickom vzdelávaní veľký význam. V súčasnosti existuje množstvo softvérov, pomocou ktorých môžu učitelia rozvíjať schopnosť vizualizácie u študentov. V článku uvádzame niekoľko dynamických pracovných listov z matematickej analýzy vytvorených pomocou softvéru GeoGebra. Zamerali sme sa na učivo poslucháčov Slovenskej poľnohospodárskej univerzity v Nitre.
Kľúčové slová: GeoGebra, dynamické pracovné listy, matematická analýza
Summary. Visualizations of study materials have a great importance in mathematical education. Today there are many types of software whereby teachers can develop the student's ability to visualize. Paper brings some of the dynamic worksheets created using mathematical software GeoGebra. We have focused on educational themes of students at the Slovak University of Agriculture in Nitra.
Back
Konstrukční úlohy
ve 3D systémech dynamické geometrie
Šárka Gergelitsová
MFF UK v Praze, Malostranské nám. 25, 118 00 Praha 1, ČR
e-mail: sarkagbn.cz
Abstrakt: Příspěvek uvede příklady úloh, které do středoškolské geometrie dosud patřily spíše okrajově – konstrukčních úloh v prostoru. S využitím systémů 3D dynamické geometrie můžeme snadno získat obrazy sestrojených útvarů a tak vizuálně ověřit zvolené řešení. Uvedeme několik úloh o mnohostěnech a o kulové ploše, ukážeme užití kulové inverze
Klíčová slova: stereometrie, konstrukční úlohy v prostoru, čtyřstěn, kulová plocha, kulová inverze, systémy dynamické geometrie, Cabri 3D
Summary. 3D dynamic geometry systems allow us to substitute time-consuming drawing by direct modeling. Systems provide immediate visualization of the constructed objects. Tasks, though, must be chosen carefully to avoid confusing scenes that may cause a loss of students’ motivation.
Back
Kudy vede cesta (k prostorové představivosti)
Šárka Gergelitsová1, Tomáš Holan2
MFF UK v Praze, Malostranské nám. 25, 118 00 Praha 1, ČR
1e-mail: sarkagbn.cz
2e-mail: Tomas.Holanmff.cuni.cz
Abstrakt. Využití počítačové hry nejen přináší do výuky oživení, ale vhodně zvolená hra může rozvíjet požadované schopnosti žáků. Testovali jsme vliv počítačové hry, která vyžadovala soustředění na pohyb v prostoru, na rozvoj prostorové představivosti žáků a jejich schopnost řešit prostorové kvízy. Test v prvním ročníku gymnázia pozitivní vliv hry potvrdil.
Klíčová slova: počítačová hra, virtuální scéna, pohyb v prostoru, prostorová orientace, test představivosti, statistické vyhodnocení
Summary. Computer games can be a useful tool in the didactic process and an easy and attractive way for training particular abilities. Tests we made with high school students showed that the game had brought benefits for the development of their spatial imagination.
Back
Visualization of Dense Placements Based
on Arbitrary Rigid Motions in the Plane
Ján Karahuta
FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
e-mail: jan.karahutafmph.uniba.sk
Abstract. The aim of the paper is to show possibilities for visualization of the set of dense placements of plane figures with respect to all rigid motions in the Euclidean plane.
Keywords: Geometric figure, set of dense placements, rigid motion in the Euclidean plane.
Back
Kružnicová inverzia a Steinerov reťazec
Mária Kmeťová
Katedra matematiky FPV UKF v Nitre, Tr. A. Hlinku 1, 949 74 Nitra, SR
e-mail: mkmetovaukf.sk
Abstrakt. V článku pomocou kružnicovej inverzie ukážeme niektoré vlastnosti takzvaného Steinerovho reťazca a riešenie úloh podobného charakteru. Pri konštrukciách využijeme dynamický program GeoGebra.
Kľúčové slová: kružnicová inverzia, dynamický program, Steinerov reťazec
Summary. The paper presents proofs of some interesting properties of the Steiner´s ring and there are solved related problems using circle inversion.
Back
GeoGebra vo vyučovaní kružnicovej inverzie
Mária Kmeťová
Katedra matematiky FPV UKF v Nitre, Tr. A. Hlinku 1, 949 74 Nitra, SR
e-mail: mkmetovaukf.sk
Summary. Using dynamic geometric software GeoGebra there are investigated properties of circle inversion and solved problems leading to illustration of Steiner´s porism.
Back
Softvér GeoGebra ako nástroj dynamickej matematiky
pre sekundárne vzdelávanie
Iveta Kohanová1, Michaela Regecová 2
FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
1e-mail: kohanovafmph.uniba.sk
2e-mail: regecovafmph.uniba.sk
Abstrakt. Školská reforma na Slovensku priniesla okrem iného i nový pohľad na vyučovanie matematiky, čo sa prejavilo na základných i stredných školách zmenami v obsahovej náplni predmetu matematika. Zmenou však prechádza i samotné vzdelávanie pedagogických zamestnancov. V článku ponúkame základné informácie o pripravovanom kurze pre učiteľov matematiky v rámci ich kontinuálneho vzdelávania. Tento kurz je zameraný na aplikáciu voľne dostupného softvéru GeoGebra do vyučovania matematiky pre sekundárne vzdelávanie.
Kľúčové slová: voľne dostupný softvér GeoGebra, kontinuálne vzdelávanie pedagogických zamestnancov, sekundárne vzdelávanie, pracovné listy
Summary. Latest school reform in Slovakia brought besides other things also changes in philosophy of teaching mathematics at secondary schools, which has influenced the changes in mathematics‘ curriculum. The change is also concerning teachers and their continual education. In this paper we speak about course for mathematics teachers that will be offered by the Methodical Centre in Bratislava. This course will focus on application of free software GeoGebra in mathematics education at secondary schools.
Back
Variational Design and Rendering
of Global Beta-Spline Curves
Soňa Kudličková
FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
e-mail: kudlickovafmph.uniba.sk
Abstract. Mathematical background for computing Beta-spline curves, their variational design with parameters bias and tension and Farin-Boehm construction for rendering Beta-spline segments via Casteljau algorithm.
Keywords: Geometric continuity conditions, Bezier curve, Beta-spline curve, blending functions, variational design, rendering.
Back
Súčet druhých mocnín vzdialeností medzi vrcholmi konvexného n-uholníka
Pavel Novotný
KKMAHI, FPEDaS, Žilinská univerzita, Univerzitná 1, 010 26 Žilina, SR
e-mail: pavel.novotnyfpedas.uniza.sk
Abstrakt: Práca podáva ďalšie zlepšenie dolného odhadu pre súčet druhých mocnín vzdialeností medzi vrcholmi konvexného n-uholníka s jednotkovým obvodom.
Kľúčové slová: vzdialenosť, konvexný n-uholník
Summary. We give another improvement of the lower bound in the inequality of G. Larcher and F. Pillichshammer for the sum of squared distances between vertices of a convex polygon with unit perimeter.
Back
Vizualizácia funicií komplexnej premennej
na Riemannovej sfére
Miroslava Valiková1, Pavel Chalmovianský2
KAGDM, FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
1e-mail: miroslava.valikovafmph.uniba.sk
2e-mail: pavel.chalmovianskyfmph.uniba.sk
Abstrakt. Ciel’om našej práce je zostavit’ sadu vizualizačných metód, ktoré umožnia skúmat’
štruktúru daného holomorfného zobrazenia určitého typu. V našom štúdiu pracujeme s rozšírenou
Gaussovou rovinou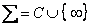 
. Pre lepšiu vizualizáciu zobrazujeme rozšírenú komplexnú
rovinu pomocou stereografickej projekcie na Riemannovu sféru, ozn. S2.
V našej práci skúmame zobrazovanie elementárnych funkcií. Jedným z prostriedkov
vizualizácie je použitie Möbiovej transformácie, ktorú aplikujeme v definičnom obore funkcie
alebo v obore hodnôt funkcie. Naša metóda súˇcasne vizualizuje argument aj modul komplexného
čísla. Argument zobrazujeme pomocou farebného kódu a transformovaná hodnota modulu komplexného
čísla určuje vzdialenost’ polopriesvitnej vrstvy od Riemannovej sféry.
Kľúčové slová: funkcie komplexnej premennej, Riemannova sféra, vizualizácia komplexných
zobrazení
Summary. The aim of this work is to compile a set of visualization methods which allow to examine
the structure of holomorphic function of a certain type. In our study, we work with extended
Gaussian plane 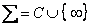. For better visualization, we display the compactification of
complex plane to Riemann sphere by stereographic projection.
We study visualization of elementary complex functions. One of the means of visualization
is the usage of Möbius transformation which is applied in the domain or values of the
function. Our method simultaneously visualizes the argument and the module of complex number.
We visualize the argument on the Riemann sphere by color transition. The value of the
module of the complex number determines the distance between the semi-transparent layer and
the Riemann sphere.
Back
Minkowského rozdiel v modelovaní plôch
Daniela Velichová
SjF STU Bratislava, Nám. slobody 17, 812 31 Bratislava, SR
e-mail: daniela.velichovastuba.sk
Abstrakt: V článku sú prezentované niektoré známe definície Minkowského rozdielu bodových podmnožín euklidovského priestoru a uvedené sú niektoré základné vlastnosti tejto operácie. Na konkrétnych príkladoch sú porovnané výsledky získané použitím jednej z definícií pri modelovaní listov plôch ako Minkowského rozdielov, súčtov a súčinov bodových množín určených vektorovými rovnicami.
Kľúčové slová: Minkowského rozdiel, súčet a súčin, modelovanie listov plôch
Summary. Several known definitions of the Minkowski difference of point sets in the Euclidean space are presented in this paper, together with some of the basic properties of this operation. Some examples are introduced, in which the results of a particular Minkowski difference definition application and Minkowski sum and product of point sets are compared, used in modelling of surface patches from curve segments determined by vector representations.
Keywords: Minkowski difference, sum and product, modelling of surface patches.
Back
Three Types of Monotone Bodies in the Euclidean
Plane and their Mutual Relations
Peter Zahradník
FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
e-mail: zahradnikfmph.uniba.sk
Abstract. By the systematic building of the theory of monotone bodies’ placements, three different definitions of monotonicity occurred in our former works. However, each of them describes a different set of geometric bodies. The aim of the present paper is to show that if we add some additional conditions to one definition then it becomes equivalent to the other.
Keywords: Geometric body; boundary, vertical and pathwise monotonicity; set of dense placements.
Back
Věty o trojúhelníku, čtyřstěnu a simplexu
Svatopluk Zachariáš
Plzeň, ČR
e-mail: svataludaseznam.cz
Abstrakt. V článku je uvedeno a dokázáno zovšeobecnění některých známých vět platných pro trojúhelníky v E2 na n-rozmerné simplexy v En, pro n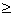 
2.
Klíčová slova: kosinová věta, Pythagorova věta, Eulerova přímka
Summary. In this paper we present some generalisations of known relations valid for triangles in E2 extended to n-dimensional simplexes in En, for n 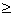2.
Keywords: Cosine theorem, Pythagorean theorem, Euler line.
Back
Pseudo-Euclidean Spaces and Hyperbolic Geometry
Pavel Chalmovianský
KAGDM FMFI UK, Mlynská dolina, 842 48 Bratislava, SR
e-mail: pavel.chalmovianskyfmph.uniba.sk
Abstract. We consider a classical topic in geometry – the space Rn with a non-singular
quadratic form with signature (n – p; p). We recall some ideas behind the structure of the
automorphisms of such space and we also illustrate the geometry of the automorphisms. These
notions are often used in various fields such as special theory of relativity, hyperbolic geometry,
geometry of Pythagorean hodograph curves and others.
Keywords: Minkowski space, Poincaré group, hyperbolic geometry, visualization.
Back
p-číslo trojuholníka je aspoň 4
Viera Čmelková1, Vojtech Bálint2
FPEDAS ŽU, Univerzitná 1, 010 26 Žilina, SR
1e-mail: viera.cmelkovafpedas.uniza.sk
2e-mail: vojtech.balintfpedas.uniza.sk
Abstrakt. Náčrt dôkazu tvrdenia P(T) 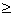4.
Kľúčové slová: p – číslo, dláždenie, trojuholník
Summary. Partridge number of arbitrary triangle is at least 4.
Back
